Figure 9. Eggs come in all kinds of shapes and sizes.

Eggs vary in origin, color, size and shape: there are bird eggs and snake eggs, white eggs and eggs in every other color of the rainbow, large and heavy eggs like Ostrich eggs and Hummingbird eggs weighing about half a gram, and round eggs and eggs with conical tops. See Figure 9 for a variety of sizes and shape. This image has been taken from Eggs − A Virtual Exhibition of the Provincial Museum of Alberta in Canada. At this website are various picture of birds eggs made available.
Figure 9. Eggs come in all kinds of shapes and sizes.

Via the algebraic and geometric approach all kinds of mathematical egg shapes have been invented (see for example the web page www.mathematische-basteleien.de/eggcurves.htm. There are many options: a piecewise defined egg curve can be constructed in many ways from circular arcs, square root curves, a parabola or by whatever function that you think matches with part of the cross sectional view on the egg. The resulting shape looks in most cases like an egg curve, but when applied to one or another egg one definition is more appropriate than another. Mathematical modeling is no alternative to empirical science. The following example illustrates this.
The following mathematical function originates from a Dutch mathematics textbook, in particular from an exercise about the volume of a volume of revolution (Staal et al., 2001, exercise 5.6.3):
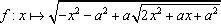
The curve y = f(x) is drawn in Figure 10 with the help of Geogebra for a = 6.1 with the hen’s egg as background image. Because of the orientation of the egg on the image I have plotted f(-x) and -f(-x) for negative x-values. The curve fits less good than the previous approximation with two ellipses. But the choice for this mathematical function was probably driven by the condition that a computer algebra system can determine the integral linked to the volume computation. For who is interested in this, the volume is equal to:
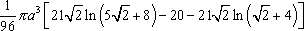
This formula specializes to 77.3 ml for a = 6.1.
Figure 10. A less suitable mathematical egg curve.
There are plenty of geometric constructions of egg curves. For example, in (Ernst, 2000) and (Klingens, 2000) a construction of the egg curve with four circular arcs has been described. Another nice example of a geometric construction that works fine for our hen’s egg is the Cartesian oval. This curve, invented by René Descartes (in Latin: Renatus Cartesius) in 1637, is the locus of points for which the weighted sum of the distances to two fixed points or foci is constant. This curve can be constructed in GeoGebra on top of a background picture of the hen's egg, as shown in Figure 11.
Figure 11. The Cartesian oval as egg curve.
The drawing in Figure 11 can be constructed in the following way: Consider the locus of points P for which
m·d(P,F1) + d(P,F2) = c,
where m and c are constants, and where d(P,F1) and d(P,F2) represent the distances of the point P to the foci F1 and F2. As origin of the coordinate system you choose the point F1. In Figure 11 you see two slider bars for the parameters m and c, respectively. In addition there has been constructed a line segment AB with a point C on it. The next thing to do is to construct a circle with center F2 and radius equal to the distance d(A,C) from A to C, and a circle with center F1 and radius equal to d(B,C) / m. The intersection points P and Q of the two circles satisfy by construction the property that characterizes a point on the Cartesian oval. This curve is drawn when you let GeoGebra determine the locus of these points that depend on the point C. The reader is however warned that there can be holes in the curve created due to numerical precision.
The Cassini oval, which is defined as the locus of points for which the weighted product of the distances to two fixed points or foci is constant, is often used to create egg curves too. But this mathematical curve does not describe well the given hen’s egg.