in the upper right corner of the drawing pad.Figure 4 is a dynamic GeoGebra figure in which
the egg curve is successfully approximated with two ellipses that are smoothly connected.
You can replay or step through the geometric construction, or you can even give it a try yourself after rewinding
the construction to its first step. You can always reset the construction to its original state by pressing the
reset icon in the upper right corner of the drawing pad.
Figure 4. A geometric approximation of the egg curve with two ellipses.
Let us dwell upon the way the ellipses in Figures 3 and
4 have been constructed.
First you need to know that an ellipse is a special case of a conic section. A conic section can be constructed in
GeoGebra by selecting the corresponding tool button 
, followed by clicking five distinct points of which no four points lie on a straight line.
Depending on the choice of the five defining points you get a hyperbola, ellipse, parabola or a special case
like a circle or a pair of intersecting straight lines. If you select five points on the egg curve,
then you get an ellipse. A good approximation of the egg curve with a single ellipse is not possible, but as you can see
in Figures 3 and 4
it goes well with two ellipses (Note: the situation does not fundamentally change in a perspective view on the
egg: two conic sections still suffice).
In the above construction of the two ellipses, two common points on the egg curve have been chosen, namely at the two spots (labeled B and C) where the egg is widest as observed with the naked eye. The coordinate system can be positioned such that these two specially chosen points lie on an axis at equal distance from the origin. The axes are scaled such that they match the scaling of the graph paper. Note that I have customized the GeoGebra applet parameters and the toolbar such that the tool button for moving and zooming of the drawing pad is not available and mouse dragging of the whole drawing pad is disabled in order to avoid accidental change of the coordinate system. This is a feature of GeoGebra for educational use: it allows the instructor to provide a GeoGebra worksheet in any specific format that is considered suitable for the students. In GeoGebra, the mathematical formula of the ellipses is shown in the algebra window. In Figure 3 you may have noticed that the formulas of the two ellipses are not in canonical form; in Figure 4 the defining points have been moved by trial and error to such positions that the equations of the ellipses have canonical form.
Bringing the points in such positions that they result in mathematical formulas for the ellipses that are in canonical form is not an easy job to do. In Figure 4 I have actually supported this by way of construction. First I have created two points on the x-axis at equal distance from the origin via an auxiliary construction of a circle with center at the y-axis. By changing the radius and/or center of this circle I can bring the two intersection points on the x-axis closer to or further away from each other while maintaining the property that they are at equal distance from the origin. Another point on the egg curve used for the construction of a particular ellipse is mirrored in the y-axis and finally a fifth point is chosen such that the mathematical formula for the ellipse becomes canonical, i.e., of the simplest form. The formulas for the big and small ellipse are
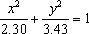
and
,
respectively. These formulas are also displayed in a nicely formatted way in the drawing pad in appropriate colors.