In the group Hybrid atom-ion quantum systems, we study the quantum physics of interacting atom and ions.
Ultracold atom-ion mixtures
Experiments with ultracold atoms and ions allow us to study
quantum phenomena in the laboratory, such as Bose-Einstein condensation,
quantum entanglement and quantum phase transitions. Although a lot of
work has been done on the systems of ions and atoms separately,
combining the two and studying their interactions in the quantum regime
is a relatively new field [1-3]. In our experiment, we study the
interactions between Yb+ ions and an ultracold gas of
fermionic Li atoms. Our experiment combines two species of atoms and
ions that have a very large mass ratio (~29). It has been shown
theoretically [4] that such a combination should allow reaching lower
temperatures such that quantum phenomena can be studied. In 2019, we
have observed interactions between a trapped ion and a cloud of atoms at
a temperature where quantum effects start to dominate [5]. To tune the
interactions between atoms and ions, we can make use of lasers that
promote the atoms to high lying Rydberg states which have a much larger
polarizability then ground state atoms and are therefore interacting
much more strongly with the ions. We have observed interactions between
Rydberg atoms and ion in 2018 [6].
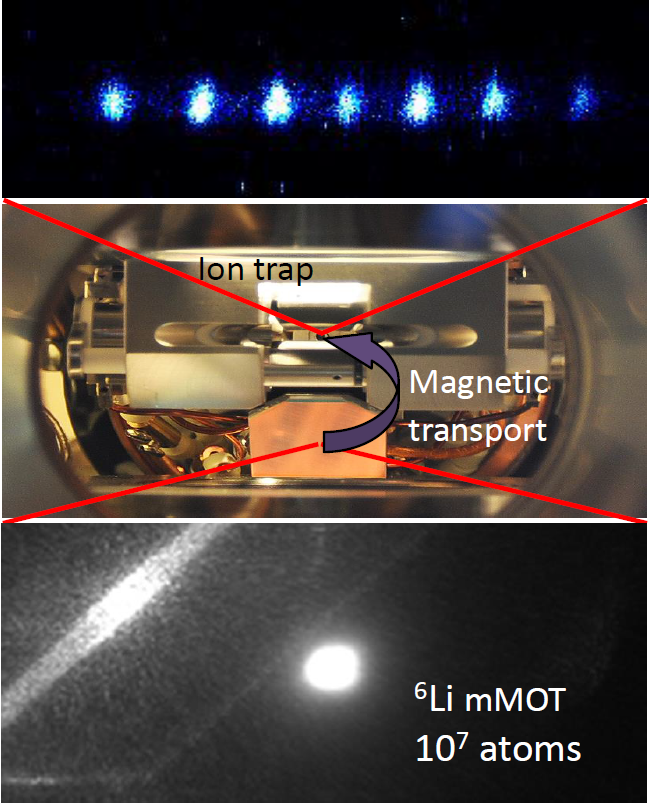
Figure: In our experimental setup, we trap crystals of Yb+ ions (top) in a Paul trap (middle). An atomic cloud is prepared a few cm away from the ions in a magneto-optical trap. Next we transport the atoms up by switching magnetic fields and let the atoms interact with the ions. In the near future, we will upgrade our atom trap to a purely optical one, allowing us to reach much lower temperatures.
Quantum simulation
Quantum simulation was proposed by Feynman in the 80s as a means to solve the problem of simulating quantum mechanics on classical computers. The problem is that the complexity of quantum problems grows exponentially with system size, such that numerical simulations of hard problems on computers become utterly impossible for systems larger than a few tens of particles. Instead Feynman proposed to employ well-controlled quantum laboratory systems (that inherently follow the laws of quantum mechanics) to mimic the dynamics of quantum model Hamiltonians of interest. This may sound a bit complicated, but actually simulators of classical physics are very common in science and technology: You can think of analogue versions such as crash test dummies or wave simulators, or digital versions such as weather forecast software, or software for predicting the movement of planets. A quantum simulator is the quantum analogue of such devices.
Since our atoms are indeed fermionic, the atom-ion mixture as stunning similarities to a natural crystalline solid (with now the atoms playing the role of the electrons) and should feature solid state phenomena such as fermion-phonon coupling and quantum phase transitions [7]. This could allow us to use the system as a quantum simulator of solids and to study the dynamics of many-body atom-ion systems.
For an overview of the field of hybrid atom-ion systems you can have a look at this review paper.
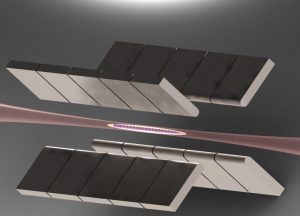
Figure: An ion crystal in a Paul trap is overlapped with a cloud of ultra-cold Li atoms trapped by a laser beam.
Trapped ions in optical tweezers
The key to quantum technology is to gain control over materials at the quantum level. The system that has reached the highest level of quantum control is without doubt ions in Paul traps. Two-qubit quantum gates have been demonstrated with errors below 0.1% [8,9] and quantum simulators containing up to 53 ions [10] have been reported.
Precision quantum gates between ions are mediated by soundwaves in the ion crystal. In the positively charged crystal, no screening occurs and the interactions between the qubits are long-ranged. This allows for very efficient implementations of quantum algorithms [11], as long as the desired qubit couplings can be distilled from the all-to-all interactions. In our experiment, we aim to implement a new way to control the interactions between the qubits allowing direct engineering of the soundwaves that mediate the interactions between the ion-qubits: To mold them into a form that exactly gives the desired qubit-qubit interactions.
The scheme uses optical tweezers that will be generated with spatial light-modulators and acousto-optical deflectors. The tweezers pin down individual ions in the crystal. Remarkably, pinning one ion changes the soundwave spectrum of the whole crystal due to the Coulomb interactions [12]. In this way, we can engineer the soundwave spectrum such that the resulting qubit interactions match the ones desired. The scheme could allow the single step implementation of the N-qubit Toffoli gate, an important gate in quantum error correction [13]. The idea is particularly promising for quantum simulation in 2-dimensional ion crystals. As a prominent example, we showed in theory [14] that the technique allows to quantum simulate a Hamiltonian that supports a spin liquid ground state in the thermodynamic limit.
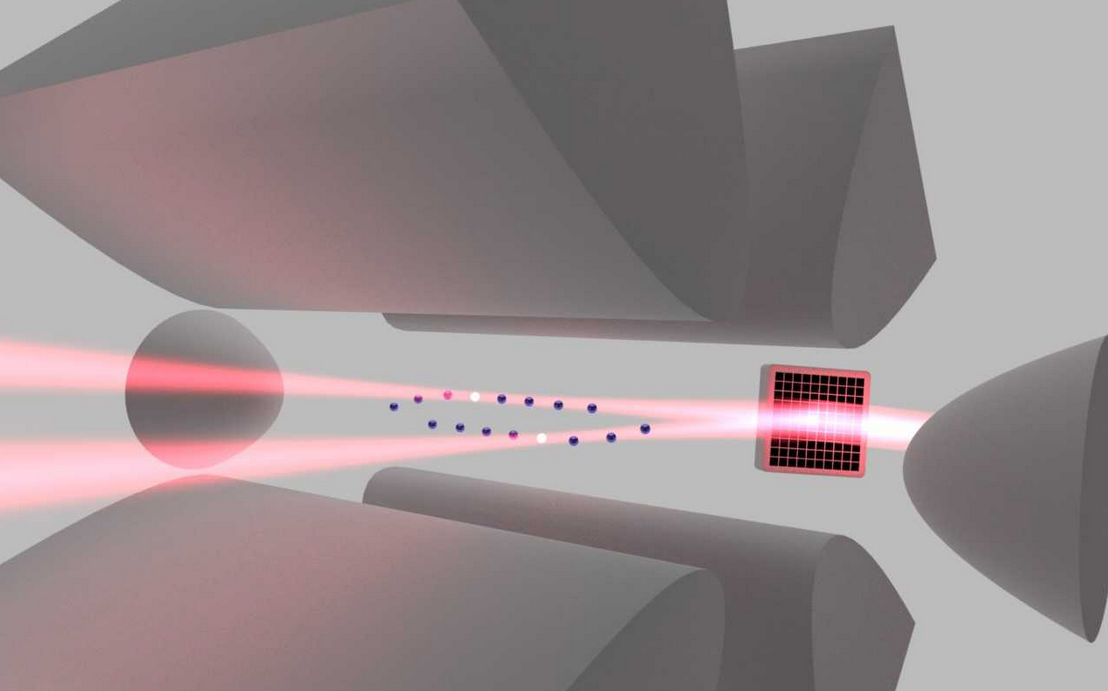
Figure: A two-dimensional ion crystal is held in a Paul trap, with each ion encoding a qubit. Optical tweezers allow for engineering the soundwave modes in the crystal, and thereby the interaction between the qubits.
Literature
[1] A. Grier, M. Cetina, F. Orucevic and V. Vuletic, Phys. Rev. Lett. 102, 223201 (2009).
[2] Christoph Zipkes, Stefan Palzer, Carlo Sias & Michael Köhl, Nature 464, 388-391 (2010).
[3] Stefan Schmidt, Arne Härter and Johannes Hecker Denschlag, Phys. Rev. Lett. 105, 133202 (2010).
[4] M. Cetina, A. Grier and V. Vuletic, Phys. Rev. Lett. 109, 253201 (2012).
[5] T. Feldker et al., Nature Physics 16, 413–416 (2020).
[6] N. V. Ewald et al., Phys. Rev. Lett. 122, 253401 (2019).
[7] U. Bissbort et al., Physical Review Letters 111, 080501 (2013).
[8] C. J. Ballance et al., Phys. Rev. Lett. 117, 060504 (2016).
[9] J. P. Gaebler et al., Phys. Rev. Lett. 117, 060505 (2016).
[10] Zhang et al., Nature 551, 601 (2017).
[11] N. M. Linke et al., PNAS 114, 3305 (2017).
[12] Lauprêtre et al., Phys. Rev. A 99, 031401(R) (2019).
[13] S. E. Rasmussen et al., Phys. Rev. A 101, 022308 (2020).
[14] R. Nath et al., New J. Phys. 17, 065018 (2015).
Funding
Our funding is provided by the European Research Counsil via the ERC Starting Grant Hybrid atom-ion Quantum Systems and the Dutch Science Foundation via a Vidi grant, a Startup grant, a Projectruime grant, and the Vrije Programma Atomic Quantum Simulators 2.0.

