Highlights

Search for broad Feshbach resonances in the ⁶Li-⁴⁰K mixture - August (2009)

We explore the widths of interspecies Feshbach resonances in a mixture of the fermionic quantum gases ⁶Li and ⁴⁰K. Experimentally, we obtain the asymmetric lineshape of the interspecies elastic cross section by measuring the distillation rate of ⁶Li atoms from an optically-trapped ⁶Li/⁴⁰K mixture as a function of magnetic field. This provides us with the first experimental determination of the width of a resonance in this mixture, ΔB=1.5(5) G, being one of the broadest. Our results offer good perspectives for the observation of universal crossover physics in this mixture.

Figure (left): The width and position of all s-wave Feshbach resonances in stable two-component ⁶Li/⁴⁰K mixtures below 500 G as calculated with the Asymptotic Bound-state Model.
Figure (right): Measurement of the Feshbach resonance width. The red solid line indicates the best fit obtained for B₀=114.47(5) G and ΔB = 1.5(5) G. The gray shaded area indicates the combined error in B₀ and ΔB.

Further reading

Broad Feshbach Resonance in the ⁶Li-⁴⁰K mixture
T.G. Tiecke, M. Goosen, A. Ludewig, S.D. Gensemer, S. Kraft, S. J. J. M. F. Kokkelmans, and J.T.M. Walraven,
Physical Review Letters 104 (2010) 053202 (PDF)

Clean Bright and Cold: a 2D MOT source for cold Lithium atoms - May (2009)

We demonstrate a novel 2D MOT beam source for cold 6Li atoms. The source is side-loaded from an oven operated at temperatures in the range 600 < T < 700 K. The performance is analyzed by loading the atoms into a 3D MOT located 220 mm downstream from the source. The maximum recapture rate of ~10⁹ /s is obtained for T=700 K and results in a total of up to 10¹⁰ trapped atoms. The recaptured fraction is estimated to be 30(10)% and limited by beam divergence. The most-probable velocity in the beam (α_z) is varied from 18 to 70 m/s by increasing the intensity of a push beam. The source is quite monochromatic with a full-width at half maximum velocity spread of 11 m/s at α_z = 36 m/s, demonstrating that side-loading completely eliminates beam contamination by hot vapor from the oven. We identify depletion of the low-velocity tail of the oven flux as the limiting loss mechanism. Our approach is suitable for other atomic species.

Further reading

A high-flux 2D MOT source for cold lithium atoms
T.G. Tiecke, S. Gensemer, A. Ludewig, and J.T.M. Walraven,
Physical Review A 80 (2009) 013409 (PDF)

Interspecies Feshbach Resonances in a Fermi-Fermi Mixture - February 5 (2008)

In a close collaboration with the experimental group in Innsbruck (Austria) and theory groups at NIST/Gaithersburg (USA) and the Eindhoven University of Technology (The Netherlands) we explored the interaction properties of ⁶Li-⁴⁰K mixtures.

Feshbach resonances appear if two elastically colliding atoms get temporarily trapped in their joint molecular structure. In traditional collision experiments these resonances are excited by variation of the collision energy. In quantum gases Feshbach resonances can be induced by changing the internal (hyperfine) structure of the atoms with a magnetic field. In figure 1 the corresponding Zeeman shifts are shown for the energy levels of the ⁶Li-⁴⁰K atom-pair at large separation (blue line) and for some relevant molecular levels (red and green lines). Whenever the blue curve crosses with one of the green or red curves a resonance is predicted. By varying the singlet and triplet binding energies the predicted resonance positions can be fitted to the experimental results obtained in Innsbruck. Interestingly, if the molecules are asymptotically bound (see figure 2) the shifts can be obtained without calculating even a single wavefunction. In this way hundreds of resonances are predicted.

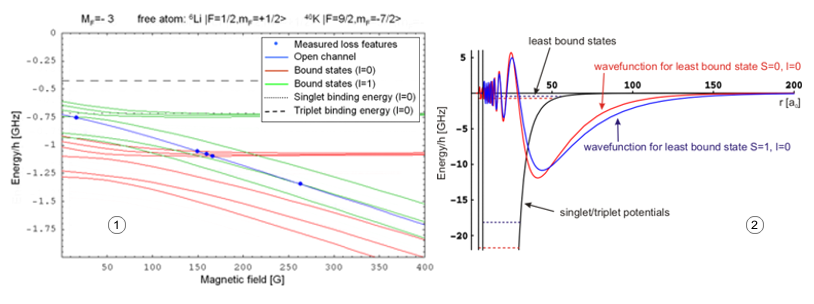Figure 1: Bound state energies versus magnetic field. Red (green) lines indicate the s-wave (p-wave) states. The two-body threshold for the Li|1/2,1/2>K|9/2,-7/2> collision channel (M_F=-3) is indicated by the blue line. The dots indicate the measured resonance positions.
Figure 2: The asymptotic bound states for the ⁶Li-⁴⁰K pair corresponding to the least bound levels. The red (blue) curves correspond to the s-wave singlet (triplet) levels and wavefunctions.

Further reading

Exploring an Ultracold Fermi-Fermi Mixture: Interspecies Feshbach Resonances and Scattering Properties of ⁶Li and ⁴⁰K
E. Wille, F. M. Spiegelhalder, G. Kerner, D. Naik, A. Trenkwalder, G. Hendl, F. Schreck, R. Grimm, T. G. Tiecke, J. T. M. Walraven, S. J. J. M. F. Kokkelmans, E. Tiesinga, and P. S. Julienne,
Physical Review Letters 100 (2008) 053201 1-4 (PDF)
Links:
Innsbruck group homepage: www.ultracold.at and IQOQI
Amsterdam group homepage: Quantum Gases Group
Eindhoven group homepage: Theory group of Atomic gases with strong interactions
NIST group homepage: Quantum Processes and Metrology Group

Shape oscillations in non-degenerate Bose gases - May 5 (2005)

The hydrodynamic flow of classical fluids was described as early as 1755 by the equation of motion of Euler. The opposite limit of collisionless flow is equally well understood since the work of Maxwell and Boltzmann and the investigation of rarefied gas dynamics around the turn of the last century. The transition regime between collisionless and hydrodynamic conditions deserves special attention as the crossover behavior is often non-intuitive as was already noted by Knudsen in 1908. With the availability of trapped ultracold gases there is a renewed interest in the collisional hydrodynamics. For non-degenerate quantum gases in harmonic traps the absence of the familiar wall-boundary condition of zero hydrodynamic flow at the sample edges gives rise to a very close phenomenological similarity with the superfluid hydrodynamics of Bose-Einstein condensates.

We have studied the crossover from collisionless to hydrodynamic conditions in non-degenerate clouds of ⁸⁷Rb by measuring both the frequency shift and the damping rates of the quadrupole shape oscillation as a function of the collisional relaxation time, which is inversely proportianal to the density. In accordance with theory, the frequency shifts up from 1.55ω_z in the hydrodynamic regime to 2ω_z for collisionless clouds, with ω_z the axial frequency of our trap. Most of the shift occurs over a narrow range of densities around the crossover density, where the mean-free-path becomes comparable to the axial size of the sample. At this density also the strongest damping is observed.

Damping rate (a) and frequency shift (b) of the quadrupole shape oscillation versus scaled relaxation time. The drawn line corresponds to the theoretical prediction for the crossover from the hydrodynamic (l.h.s.) to the collisionless (r.h.s.) regime.

Further reading

Shape oscillations in non-degenerate Bose gases - transition from the collisionless to the hydrodynamic regime,
Ch. Buggle, P.Pedri, W. von Klitzing and J.T.M. Walraven, Physical Review A 72 (2005) 043610 1-10 (PDF)

Bose-Einstein condensates in a linear accelerator - June 25 (2004)

The atomic scattering length a is the central parameter for the theoretical description of ultracold bosonic Quantum Gases. The scattering length is the elastic scattering amplitude in the zero energy limit. In all cases but hydrogen this scattering length has to be determined experimentally. In Amsterdam we developed an interference method to measure the full (complex) scattering amplitude. The method - inspired by subatomic physics - involves the acceleration of two Bose-Einstein condensates to collision energies ranging from 100 microkelvin to 1.5 millikelvin. Using computerized tomography to process the images of the scattering halos, we observe the s-d interference in the differential cross section. The accumulated phase method allows us to infer the s- and d-wave phase shifts and the s- and d-wave scattering amplitudes from the zero-energy limit up to the first Ramsauer minimum, provided the Van der Waals C₆ coefficient is known. For ⁸⁷Rb we obtain a= 102(6)a₀ for the s-wave scattering length and 300(70) microkelvin for the location of the d-wave resonance.

s-wave scattering (0.13 mK)	d-wave scattering (1.3 mK)
fields of view ~1mm (real data movies created by	Christian Buggle)

Further reading

Interferometric determination of the s- and d-wave scattering amplitudes in ⁸⁷Rb
Ch. Buggle, J. Leonard, W. von Klitzing and J.T.M. Walraven, Physical Review Letters 93 (2004) 173202.

Imaging of s- and d-wave partial wave interference in quantum scattering of identical bosonic atoms
N.R. Thomas, N. Kjaergaard, P.S. Julienne and A.C. Wilson, Physical Review Letters 93 (2004) 173201.

Tuning a Feshbach resonance through a shape resonance in the dissociation of ultracold molecules
T. Voltz, S. Durr, N. Syassen, G. Rempe, E. van Kempen, S. Kokkelmans, cond-mat/0410083.

Halo's uit botsende Bose-Einstein condensaten
Tobias Tiecke, Christian Buggle and Jook Walraven, Nederlands Tijdschrift voor Natuurkunde, 71 (2005) 208-211.

News coverage

Botsende Bose-Einstein-condensaten leveren informatie over hun interne structuur FOM-News (29 oktober 2004)

Accelerator for BECs AIP: Physics News Update (3 November 2004)