3.2 Levels of modelling
Descriptive modelling: Free fall
The first step on the road to scientific modelling is to learn how to describe a problem situation adequately with the aid of scientific symbols, mathematical relations, and graphs.1
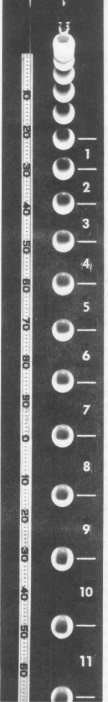
As an example of describing and predicting changes, free fall can be studied. Students carry out an experiment to determine the acceleration of free fall, for example via video measurement. Prior to the experiment, students name the relevant state variables and describe the purpose of the experiment.
The students work with a number of different objects and make a data sheet for a number of time steps Δt. From this, the acceleration of free fall is determined during each time step.
|
TIME STEP
# |
POSITION
(cm) y |
CHANGE OF
POSITION (cm) Δy |
AVERAGE
VELOCITY (cm/s) Δy/Δt |
CHANGE OF
VELOCITY (cm/s) Δv |
AVERAGE
ACCELERATION (m/s2) Δv/Δt |
| 1 |
|||||
| 2 |
|||||
| .. |
|||||
| AVERAGE OVER
TIME STEPS |
⩰ 9.81 |
||||
TABLE 1. Falling motion in time steps
The results are displayed in (y,t) en (v,t)-diagrams and the average acceleration of free fall is computed. Relevant aspects, including the dependence on the mass, the assumption that air resistance can be neglected, and the accuracy of the measurement, are discussed in the classroom.
Table 1 is a simple descriptive model of free fall that can then serve as a starting point for the development of a conceptual model consisting of variables and relations between them, a so-called dynamic model. There the transition takes place from discrete to continuous variables and time as an independent variable, plus the equations of motion are introduced. This transition is an essential step in learning modelling of dynamic processes and it is very important to pay explicit attention to this in the classroom.2,3