5.2.1. Ohm’s Law¶
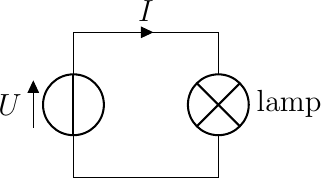
Fig. 5.7 Simple Circuit with a Battery and Lamp
Consider the simple circuit of a battery two wires and a lamp. Because of the closed circuit a current \(I\) flows, measured in Amperes (\(A\)), from the plus connection of the battery through the wire and through the lamp. The fresh battery delivers a voltage \(U\) of 1.5 Volt(\(V\)) and we assume it is constant voltage source.
How much current will flow in the circuit is determined by the total electrical resistance in the circuit. In electronics we distinguish between:
Conductors, materials that conduct electrical currents. Their resistance is most often finite (with superconductors being the exception). Metals are good conductors, as are liquids.
Insulators, materials that do not conduct. Think of wood and most plastics.
Semiconductors are somewhere inbetween conductors and isolators. But what makes semiconductors so special is that they can be doped to influence conduction. These doped semiconductors are the basis for transistors and are at the heart of any electronic circuit including computers.
As usual in electronics we follow the convention that the resistance of an electrical wire is considered negligible. So in our scheme of the battery and the lamp connected through wires the resistance is in the lamp.
A resistance \(R\) is measured in ohms (\(\Omega\)). Ohm’s law relates the voltage \(U\), the current \(I\) and the resistance \(R\):
Here we have assumed a voltage source that puts out a constant voltage that does not change in time: a DC voltage source. Then the current \(I=U/R\) is a constant current as well.